Author:
(1) Ahmed Farag Ali, Essex County College and Department of Physics, Faculty of Science, Benha University.
Table of Links
Space-time quanta and Becken Universal bound
Space-time quanta and Spectral mass gap
Conclusion, Acknowledgments, and References
III. SHAPE OF SPACE-TIME QUANTA
A natural question arises, what is the geometric shape of the space-time quanta? To answer this question, we need first to apply the energy-momentum relation that is satisfied by Snyder’s algebra [38]:

where m is the mass of the physical object. Substituting Eq. (3) and Eq. (4) into Eq. (8), we get:

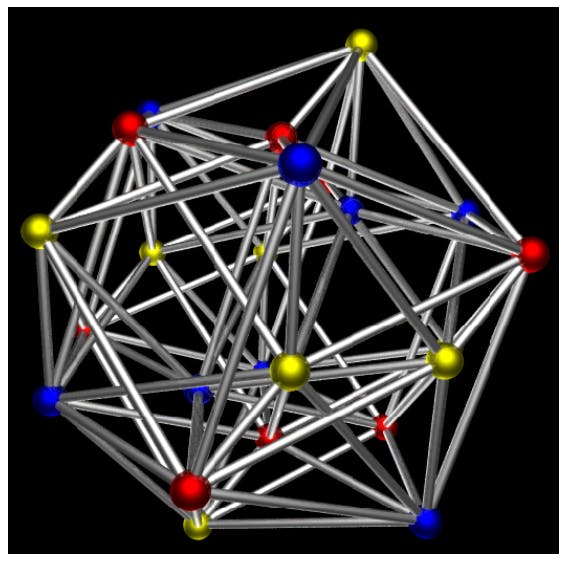
This means that the mass of the space-time quanta is completely determined by its unique length and it is strictly a “positive real value”. It is worth mentioning that generalized forms of Snyder algebra with de Sitter background imply modification of dispersion relation [39] that may introduce corrections to the mass of space-time quanta obtained in Eq.(9). The flatness of quantum Lorentzian space-time suggests that the space-time quanta be described by 4-polytope geometry [40]. We use elementary particle physics as a guide to building the quanta of space-time. The standard model of particle physics has 25 fundamental particles that include 12 fermions (quarks and leptons), 4 gauge bosons that carry electromagnetic force and weak nuclear force, 8 gluons that carry strong nuclear force, and 1 Higgs scalar field. The quanta of space-time must carry a signature of information from all these fundamental particles that constitute the fundamental structure of nature. The space-time quanta must be “self-dual” as well to preserve its uniqueness. Therefore, we look for a highly symmetric 4-dimensional geometric object that is self-dual and identified by only unique length which could represent the fundamental particles of nature on its vertices. This could be a uniform 4-polytope which is a 4-dimensional object with flat sides/faces and is vertex-transitive symmetric which means an isometric map of any vertex onto any other. A regular 4-polytope has the highest degree of symmetry as its faces/cells are regular polytopes and transitive on the symmetries of the polytope. More symmetries are found in regular 4-polytopes that define the convex region as a subset that intersects every line into a single line segment. There are two self-dual convex regular polytopes that are 5-cell, which has 5 vertices, and 24-cell which has 24 vertices. We choose the 24-cell because it has enough vertices to assign with elementary particles. A representation in a plane of a regular 24-cell is given in Fig. (1):
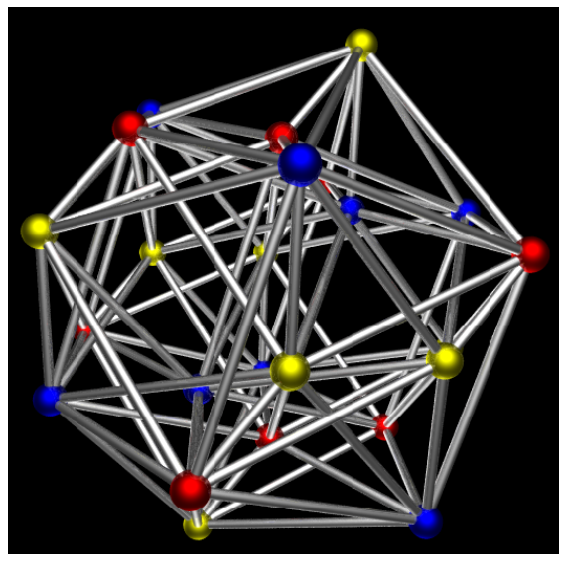
The 24-cell geometric properties can be summarized as follows:
• Its boundary in 3-dimensions forms 24 octahedral cells with six meeting at each vertex, and three at each edge
• It has 96 triangular faces, 96 edges, and 24 vertices. The vertex figure is a cube
• self-dual [41]
• identified by one length where edge length equals the distance between the center and vertex (radius)
The 24-cell exists in 4- dimensional Euclidean geometry, but Minkowski space-time is 4- dimensional “pseudo-Euclidean”. This can be simply resolved if time is represented as an imaginary spatial dimension which is well-established in quantum field theory [42] and in Euclidean quantum gravity [43]. To put it another way, the space-time quanta is represented by a 24-cell with considering time as an imaginary spatial dimension. The covariance principle requires that the space-time quanta should represent the elementary particles of the standard model [44]. In the next section, we show how to do this representation.
This paper is available on arxiv under CC BY 4.0 DEED license.